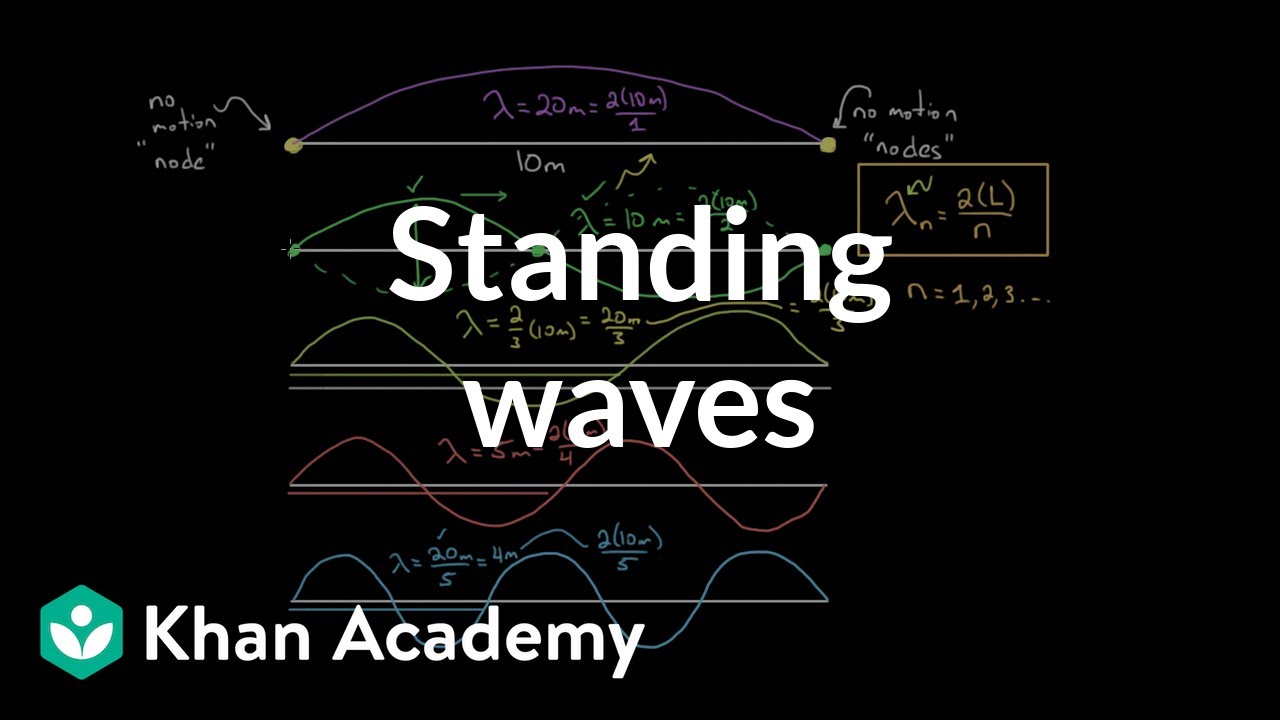
The purpose of this experiment is to investigate the behavior of standing waves caused by an external force to initiate a transverse wave on a string. Standing wave occurs when an original wave and a reflected wave superpose. Standing waves have constant wave pattern, and the amplitude of the points on the wave are changing.
- Standing Waves Lab Worksheet Answers
- Sources Of Uncertainty In A Standing Wave Experiment Youtube
- Sources Of Uncertainty In A Standing Wave Experiment Pdf
The purpose of this experimentis to investigate the behavior of standing waves caused by an external force toinitiate a transverse wave on a string. Standing wave occurs when an originalwave and a reflected wave superpose. Standing waves have constant wavepattern, and the amplitude of the points on the wave are changing. The onlypoints that are not changing in a standing wave are nodes. Node is half of thewavelength.
The relationship between the length of the string (L), number ofhalf wavelengths or loops (n), and wavelength (λ) is indicated. The experiment was conductedusing various apparatus such as variable frequency wave driver, function generator,200g and 50g weight hanger, table clamps, rod, pendulum clamp, pulley,multimeter, and a meter stick. The mass and length of the string were recordedto determine its mass density, μ. One end of the string was attached to a 200gweight hanger, and the other end was tied to the clamp. The length or distancebetween each end was measured to be 1.2m. The string wave driver which wasconnected with the function driver was put 10cm away from the pendulum clamp.The function generator was set to 5.0 voltages, and the frequency was adjustedto get a certain number of loops.
Frequencies with respective number of loopswere recorded. The procedure was repeated using 50g weight hanger in place of200g. The wavelength and velocity were computed based on the data.
Two graphswere constructed, and the velocity obtained from the graph was compared withthat from computation. As shown in table 1 and graph 1, thewave speeds from computation and from the graph were approximately 40.0 m/s and46.04 m/s. Even though these values did not agree within the uncertainties, theseresults were very similar. In same manner, the wave speeds were 20.0m/s and24.34 m/s respectively when 50g hanging mass was used. These results were shownin table 2 and graph 2. Again, these values were not within the uncertainties.
Incolumn 2 and 3 of table 1 and 2, the comparison between the recordedfrequencies and the computed frequencies were shown. The frequencies on column3 were a factor of the first recorded frequency, and these should have been thesame with the recorded frequencies. However, column 2 and 3 values were notagreed within the uncertainties.
As wavelength decreased, larger deviation fromthe recorded frequencies was observed. According to table 4, the ratios ofwave speed computed based on data and graph were approximately 2. Even thoughthe ratios were not exactly the same, they highly agreed with each other. Thisresult was confirmed by the equation 3 mentioned in the introduction. Sincevelocity was proportional to square root of tension, velocity had to decreaseby a factor of 2 when tension decreased by a factor of 4.

In table 3, it was shown that the ratio of ƒ 1and ƒ 2 were approximately 2. Even though the ratio changed a littlefor different loop numbers, the ratio maintained fairly constant. This wasreasonable because frequency and velocity were directly proportional accordingto the equation 2. Therefore, as velocity increased by a factor of 2, so didthe frequency.
There were a lot of possible experimental errors that could cause the above compared values not to agree within uncertainties. First, there was an inaccuracy in adjusting frequencies to obtain an exact number of loops. As thefrequency was raised to higher value to obtain more nodes, the wave becameharder to visible. Another factor influenced the experimental result was thatthe loop should be counted starting from the wave driver, but the loop was counted fromthe point of the clamp where the string was tied. This was shown in figure 2.
If the number of loops wascounted from the wave driver, the frequency could change. Therefore, thesefactors could contribute to inaccurate values of frequency which then led toinaccurate wave speed. In addition, the hanging mass did not remain stablethroughout the experiment.
This vibration in hanging mass could also cause avariation of tension in the string. Approximation in reading the length of thestring also contributed to these errors. All of these led to inaccuracy incalculations of wavelength and velocities.
You are given a string that is attached to a buzzer, which vibrates at a frequency 60 Hz. You can use a standard house/car key to slide along the string in order to change the length of the vibrating part of the string and find the nodes of the standing wave. The other end of the string loops over a pulley, and is attached to a hanger on which you can hang objects of different mass.
Your task is to generate two standing waves on the string; one must have a wavelength which is half that of the other one. You must predict the mass of the hanging objects necessary to do this. The first mass you hang is arbitrary. That will establish the first wavelength. Then you will need to find what mass to hang in order to get the second wavelength.
After you have made a prediction, you can perform the experiment and compare the outcome with the prediction. You are given a balance (to share), a ruler, a string, a pulley, a mass hanger, different masses, and a buzzer.Any lettered section can be graded. Items which include a letter and a number in parentheses refer to a rubric ability. For example, (T3) refers to the testing experiment rubric ability 3. Items without such designation are given as additional guidance.
(T1) Identify the hypothesis (rule) to be tested.b. (T2) Design a reliable experiment that tests the hypothesis including a brief description of your procedure.c. Construct sketches of standing waves on a string for the first two wavelengths. Keep the length of the string fixed.d. Determine a relationship between the wavelength and the hanging mass. You may consult the textbook to find relevant equations. Which parameters do you know?
Which will you need to determine?e. Choose an initial mass and find the initial wavelength experimentally.f. Devise the mathematical procedure that you will use to make your prediction for finding the standing waves.g. (T4) Make a prediction about the outcome of the experiment based on the hypothesis.h. (T5) Identify the assumptions made in making the prediction. What assumptions about the objects, interactions, and processes you need to make to solve the problem?i. (T6) Determine specifically in which assumptions might affect the prediction.j.
What are experimental uncertainties in this experiment?Perform the experiment.k. Record the outcome of your experiment.l. (T7) Decide whether the prediction and the outcome agree/disagree.m.
Decide whether your assumptions and experimental uncertainties can account for any discrepancy between the predicted and measured value.n. (T8) Make a reasonable judgment about the hypothesis based on your experimental outcomes, the assumptions you made, and the estimated uncertainty.
Find the frequencies of the first four standing waves in a sound tube (the fundamental and the first 3 overtones). You have a microphone, speaker, tube, function generator, and oscilloscope. Please keep the volume as low as possible so all stations can hear the sound in their tube. If you need help with the oscilloscope, then please ask your TA.
Before taking any data, verify that with the function generator set at 1 kHz, the corresponding wave is recorded on the oscilloscope. Hint: What is the period of a 1 kHz sine wave? Make sure the duty cycle knob on the function generator is in the calibrated position.Any lettered section can be graded.
Standing Waves Lab Worksheet Answers
Items which include a letter and a number in parentheses refer to a rubric ability. For example, (T3) refers to the testing experiment rubric ability 3. Items without such designation are given as additional guidance. (T1) Identify the hypothesis (rule) to be tested.b. (T2) Design a reliable experiment that tests the hypothesis including a brief description of your procedure.c. Determine from the physical setup if each end of the tube is a node or an anti-node of displacement of the air molecules, and explain your reasoning.
Sources Of Uncertainty In A Standing Wave Experiment Youtube
Hint: Is it an open-open, open-closed, or closed-closed tube?d. The oscilloscope reads the microphone signal, which responds to pressure. Explain how the reading on the scope relates to the oscillatory motion of the air molecules.e.
Construct sketches of standing waves in the tube for the first four standing waves.f. Mathematically determine the corresponding frequencies of the first four standing waves.g. (T4) Make a prediction about where to find the nodes based on the mathematical application of the hypothesis.h. (T5) Identify the assumptions made in making the prediction.
What assumptions about the objects, interactions, and processes you need to make to solve the problem?i. (T6) Determine specifically in which assumptions might affect the prediction.j. What are experimental uncertainties in this experiment?Perform the experiment.k. Record the outcome of your experiment.l. (T7) Decide whether the prediction and the outcome agree/disagree.m.
Sources Of Uncertainty In A Standing Wave Experiment Pdf
Decide whether your assumptions and experimental uncertainties can account for any discrepancy between the predicted and measured value.n. (T8) Make a reasonable judgment about the hypothesis based on your experimental outcomes, the assumptions you made, and the estimated uncertainty.o. If you have time remaining, then choose the n = 3 standing wave and use the string to pull the microphone through the tube to find the positions of the antinodes, and use that value to calculate the effective length of the tube, and compare that to the actual length of the tube. Which value is bigger? Why do you think that might be so?